A multipart series on the history of conquering the sound barrier, written by Dale J Ferrier

Rules are made for people who aren’t willing to make up their own.

Chuck Yeager

It’s October 1947 and in the scorching heat of the Mojave Desert, there stands a group of engineers and scientists, anxiously waiting to see if their gamble has paid off. The answer soon arrived when the pristine calm of the desert abruptly vanished with a deafening crack. Was it a disaster? An explosion? No, Chuck Yeager in his Bell X-1 had very dramatically signalled the start of the supersonic age. Getting there however was a long hard road, one festooned with triumph and tragedy. This is that story.

Just What is the Speed of Sound?

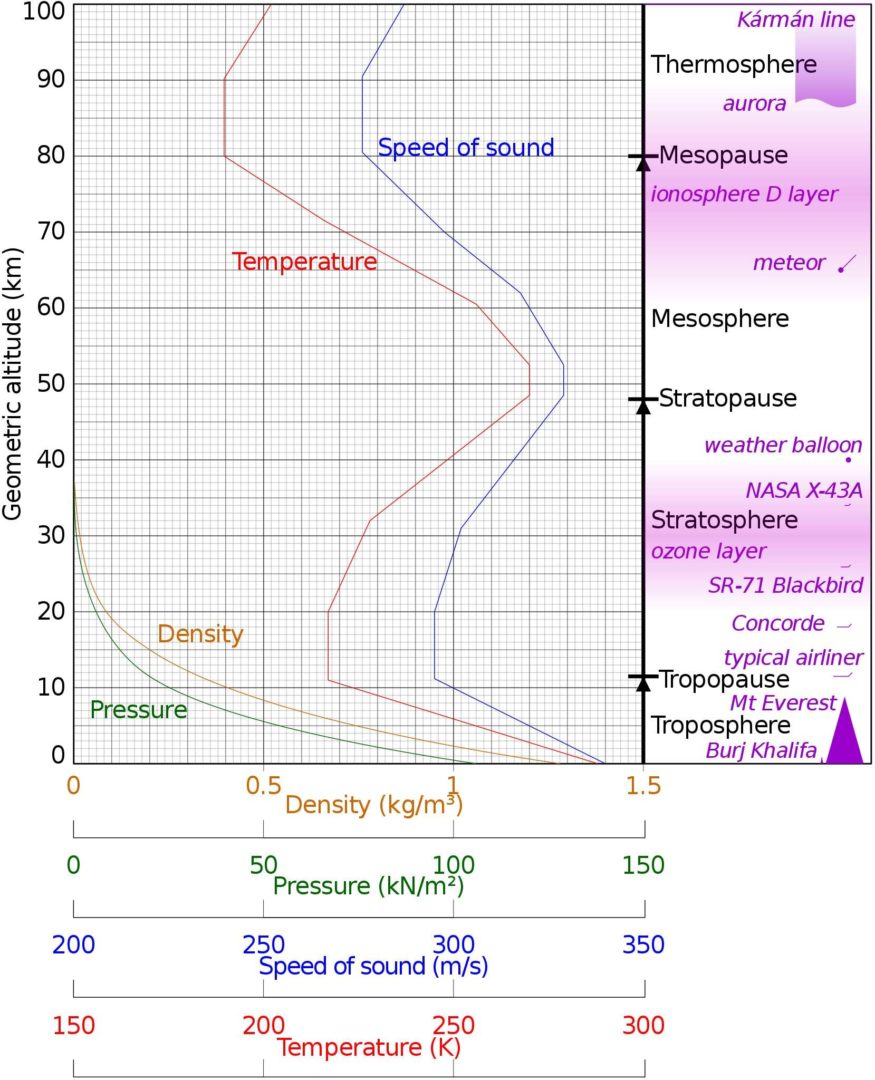
The quest for faster-than-sound manned flight would prove to be the greatest aeronautical challenge since the Wright Brothers, and the next great milestone in mankind’s endeavour to rule the heavens. But before we explore how we broke through nature’s invisible wall, we should understand what the Speed of Sound actually is.

Sound is simply when waves are transmitted through a medium by its source affecting the molecules that make up that medium, which in turn influence their neighbouring molecules. When those waves eventually influence the hairs in our eardrum, the neurological signals they create allow us to perceive them as sound, providing their frequency is within the range we can detect – which is generally between 20 and 20,000 hertz. This range varies between different species. For example dogs can detect frequencies higher than us humans.

Normally, these sounds appear to reach us instantaneously, but they do have a finite speed. It may have even occurred to our pre-historic ancestors that they saw a bolt of lightning before the resulting rumble of thunder, and the nearer they were, the quicker this thunder came. You may be familiar with the idea that we can estimate how far away the lightning was by timing how long it takes the sound of the thunder to reach us.

How quickly sounds reach us is dependent on the medium it is travelling through. The sound we hear has of course travelled through the air, even if only for part of its journey, but sound waves can indeed travel through any medium that contains some sort of mass – in space no one can hear you scream, because it’s a vacuum and so there is no mass for the sound to travel through. But, how quickly it does travel is down to two key properties; The Elastic Modulus and the material’s Density.

The Elastic Modulus is simply how compressible the material is. Rubber is pretty compressible, but certainly not as much as air! What makes a material ‘spongy’ or not depends on the bonds that exist between the molecules making it up. Each molecule forms electromagnetic bonds with other molecules, ‘sticking’ them together to form the overall material.

Gases function differently but how strongly each molecule affects another remains important and its Elastic Modulus is referred to as it’s Bulk Modulus instead. How strong these bonds are is the Elastic Modulus and stronger bonds mean that sound waves can travel from molecule to molecule faster than weaker bonds which compress more slowing the transfer of the sound waves.

Density is how much mass is contained in a defined area. A cube of air is far less dense than an equal-sized cube of steel. Sound is a form of energy and on the molecular level, it is the energy that moves these molecules back and forth in a wave. The heavier these molecules, the more energy is needed to move them to form the sound waves, and so sound is slowed down by denser materials. The effects of this can be seen right down to small differences in the atomic mass of the atoms involved. Sound will travel faster through normal ‘light’ hydrogen with its atomic mass of 1, than deuterium ‘heavy’ hydrogen with an atomic mass of 2 even though both are chemically identical.

These two factors act against each other with a higher Elastic Modulus leading to a higher speed of sound, but conversely, a higher Density lowering it. And one factor can greatly counteract the effects of the other. The speed of sound in water is around 4.3 times higher than in air, even though its Density is some 830 times greater. This is because water’s Elastic Modulus is far higher, more than overcoming the difference in Density. In gases, a change in pressure alone does not change the speed of sound as the effects of the changing Density and Elastic (Bulk) Modulus cancel each other out. The Speed of Sound does however decrease with altitude because of the reduced air temperature, not lower pressure.

Discerning that sound did indeed have a finite speed may have been made by our ancient ancestors with some Greek philosophers making vague references to it. But it would be much more recently that efforts were made to measure this speed with the earliest attempt we know of being in 1630 by Marin Mersenne who measured 1380 Parisian feet per second (A Parisian Foot is longer than a modern day Standard Foot at 325mm and 304.8mm respectively.) – about 30% too high. Robert Boyle in 1635 calculated 1,125 PF/S, and Sir Isaac Newton in his 1687 work Principa 979 PF/S.

Although respectable attempts, it was in 1709 that a more accurate measurement was made by Reverend William Derham who timed how long it took the sound of a gunshot to reach him. By using well-defined landmarks so that he knew the exact distances used and a half-second pendulum, he measured 1,072 PF/S which is remarkably close to the modern-day official speed of 1,055 PF/S (at 20C). When it comes to air moving at these speeds, or aircraft moving through the air, aerodynamicists define three distinct realms around the Speed of Sound; speeds up to Mach 0.75 are Subsonic, between 0.75 and 1.25 Transonic, and 1.25 to 5.0 Supersonic.

Going above Mach 5 is considered Hypersonic and the laws of Aerodynamics are often consumed by other phenomena such as Thermodynamics and Magnetohydrodynamics. As to where ‘Mach’ comes from, it was a name proposed by aero engineer Jakob Ackeret in 1929 in honour of Austrian physicist and philosopher Ernst Mach. And until it was exceeded, the Speed of Sound was called Mach’s Number rather than Mach 1.

The Theoretical Frontier

In 1935 during an open day at the National Physical Laboratory in the UK, the British aerodynamicist W F Hilton stood in front of a large graph displayed on the wall. It described the relationship between airfoil drag and the free stream Mach number. To those present, he said ‘See how the resistance of a wing section shoots up like a barrier against higher speed as we approach the Speed of Sound.’

The line on the graph did indeed shoot up very abruptly to what appeared infinity and although it was well-known that it had a peak, it still loomed over aero engineers as some great invisible wall that threatened to wreak havoc and destruction upon any aircraft that dared surpass it. If those engineers wished to tame that great invisible wall, they had to employ all their skill and understanding to design an aircraft that could not only be powerful enough to beat it but also survive the ordeal.

The reporters at the open day mistakenly attributed the term Sound Barrier to Hilton who only used the word to help illustrate the problem. Regardless, the term stuck and breaking through it became a holy grail for aviation. Doing so would require innovation in every aspect of flight, including the theoretical. Aerodynamicists sought to understand how air would behave around an airfoil when it approached the Speed of Sound and then passed it. Experimental data could only go so far – engineers needed to understand the mathematical framework of supersonic flight to design aircraft to reach it.

As an object moves through the air faster, the drag it experiences increases exponentially. Because of this fact, calculating the non-linear equations involved can be very complex, more so with the lack of powerful computers in the 1930s and 40s, meaning they had to be done by hand. When that object becomes supersonic, the airflow around it behaves differently than when it was subsonic, bringing about further complexities to these calculations. An example is that a disturbance in subsonic airflow can propagate upstream, but not so with supersonic flows in which the effects of the disturbance can only be seen downstream, spreading aftwards in a triangular shape from its source.

The equations for either subsonic flow or supersonic flow can be used independently when the entirety of the aircraft is fully within the realm of one or the other. However, during the transonic phase, even if the aircraft as a whole is subsonic, there will be areas experiencing supersonic flow as the air is accelerated around the airframe. This phenomenon is called an aircraft’s critical Mach number and would prove to be a potentially lethal hazard to aviators.

It is then during this phase that both kinds of non-linear equations are relevant and the associated complexities become their own mathematical sound barrier. Thankfully, when using very thin airfoils, the simultaneous use of these calculations can be simplified into approximations. But, approximations they were and although today we have a far greater understanding of this kind of transitionary airflow, even when Chuck Yeager was flying his X-1, our understanding of the transonic range was far from certain.

Breaking the mathematical enigma of transonic flight – the gateway to supersonic – took several decades of dedicated work by mathematicians and engineers from the US, Britain, France, Germany, Sweden, Russia, and Japan illustrating the international will to achieve this next great milestone. The breakthrough came three times separately when Klaus Oswalitsch and K Weighardt as well as Adolf Busemann and Gottfried Guderley, both teams in Germany, along with Theodore Von Karman in the US came on the idea of using a linear equation to bridge the gap between subsonic and supersonic flows. It was not a perfect solution, but the nonlinear small disturbance equation provided the basis for a great variety of airfoil designs at a range of Mach numbers paving the way for theory to be put into practice.

This work continued after WWII with Guderley at the Air Material Laboratory in Ohio, and Busemann at NACA’s (National Advisory Committee for Aeronautics, the predecessor to NASA) Langley Laboratory in Virginia when the pair were brought to the US under the controversial Operation Paperclip which saw over 1600 German scientists and engineers transferred to work on government research.

End of Part I